
It's the Final Countdown
By: John Vereen
By
looking at the GSP animation, it appears that Barney will always return to his
starting point during his travels. I am going to prove that, no matter where
Barney starts his travels in the triangle, he will always end up back at his starting
point when travelling on a path parallel to a side of the triangle. 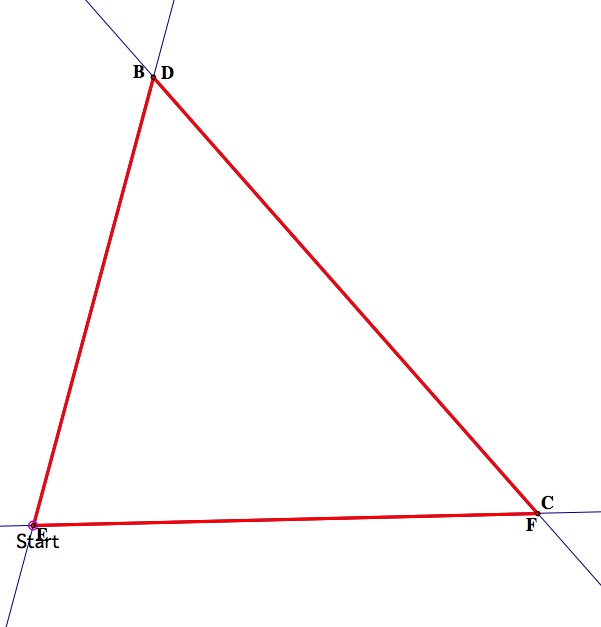

We see that there are two basic cases where Barney will surely end up at his starting point in only three steps or two walls! The first case is when Barney starts on one of the triangle vertices. This case is trivial, and does not require much of an explanation besides the fact that he walks the perimeter of the triangle. The next case we have is the case where Barney starts at the midpoint of one side. If this is the case, then Barney would walk the path of the Medial triangle because, by construction, the line parallel to one side of the triangle would have to send him to a midpoint each time. Thus, he would end up back at his starting point in only three steps .
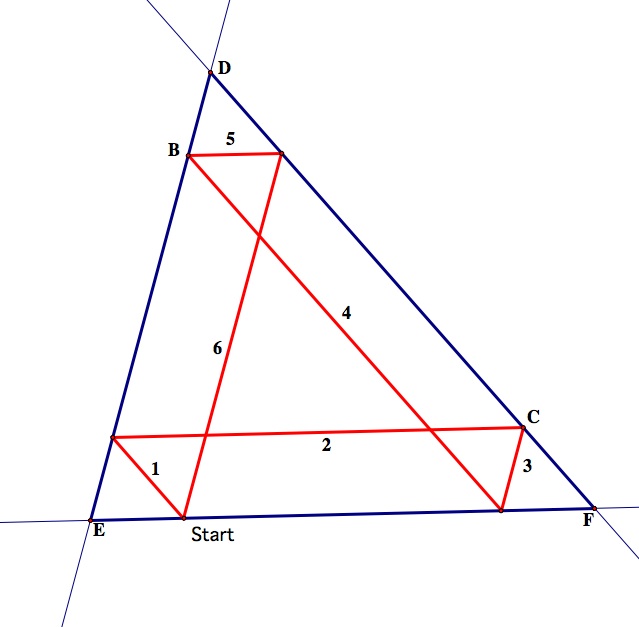
At any other arbitrary location along the segment, Barney’s path requires 6 steps (5 walls), but he still arrives back at his previous location. Why is this?
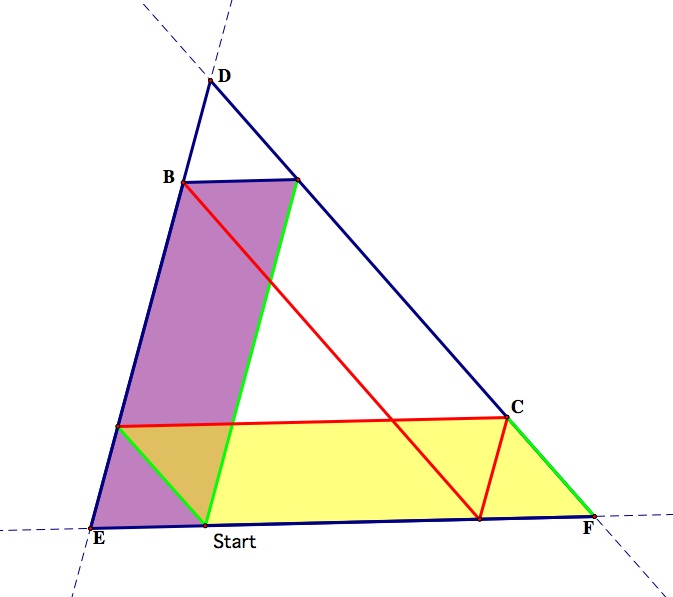
Well, we can construct 2 parallelograms (purple and yellow above) that both have vertices at Barney’s starting point. This means that each parallelogram has a segment Barney travelled (green) that share an endpoint at Barney’s starting point. This proves that, if Barney’s first step is from either of those segments, then he must come back to that point travelling by the other segment.

One question we need to answer is, “Will Barney always travel the same distance?” The answer is yes, he will always travel the same distance, and that distance, is the perimeter of the triangle. Why?
Well, the picture above proves this idea. We know that we have six steps that Barney could possibly travel from arbitrary points. We also know that parallelograms have congruent opposite side lengths. Each of the 6 steps Barney takes corresponds to a side length of a parallelogram, and these side lengths add up to the perimeter of the triangle as depicted by the color-coded sides lengths above.
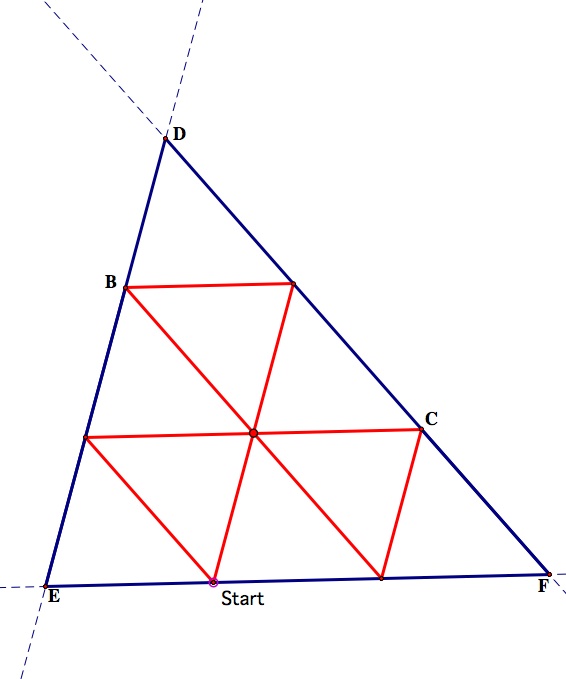
It is also interesting that, when the six paths intersect at the centroid, then it produces nine congruent triangles. We can prove this conjecture using the SAS postulate of Euclidean geometry. We can prove this by a huge series of Alternate Interior and Corresponding Angles due to parallel lines.